 Pythagoras is making musical instruments. It is carved on the main gate to the west of Sartre Cathedral. [Wikipedia]
Pythagoras is making musical instruments. It is carved on the main gate to the west of Sartre Cathedral. [Wikipedia]
The Pythagoreans considered numbers to be geometric, physical, and arithmetic entities made from dots or particles. In other words, they thought that number was the reality that made up the universe. The points of these units were placed at the vertices of various geometric shapes, and they were called triangle numbers, square numbers, etc. In the Pythagorean school, numbers have quantitative sizes and geometric shapes. It was in this sense that they understood numbers as natural forms and shapes at the same time.
Let's take a look again at how numbers connect to shapes, to the reality that makes up everything. They made numbers from individual units as if they were counting pebbles. The words calculus and calculation come from the pebbles used by the Pythagorean school. They used pebbles for counting, which represented the amount. According to the counting method, the number of one was a pebble, and the other numbers were made by adding pebbles.
However, the pebbles did not merely represent quantity. It also made a certain shape. This leads to an important fact that the Pythagorean school discovered the relationship between algebra and geometry. One pebble is one, just like one point. But the two pebbles consist of two points. The two points form one line. Like the three vertices of a triangle, three points form one plane, and four points show one cube.
These facts indicate a close relationship between number and size. Pythagoras proved that the equation we know as the Pythagorean theorem, namely, the sum of the squares of two sides perpendicular to the right triangle, equals the squares of the hypotenuse. All shapes and numbers are not abstract concepts, but concrete entities.
According to the Pythagorean school, the argument that 'all things are numbers' means that there are numbers at the foundation of all things that have shape and size. Therefore, they developed from algebra to geometry and further to the structure of reality.
All things have numbers, and their evenness or oddity explains the conflicting characteristics of objects such as one and many, square and rectangular, straight and curved, stationary and athletic. Even brightness and darkness were numerical adversaries, and so were men and women, good and evil. The Pythagorean school went so far as to form their most important philosophical concept by understanding numbers in this way. The most meaningful contribution they have made to philosophy is the concept of form.
The Pythagoreans cult worshipped number not only mathematically but also religiously. They considered 1 to be the source of all numbers. This shows that they understood some of the concepts of 'infinite'. No matter how large a given number is, they can make a larger number by simply adding one to the number. 2 is the first female number, even number, and symbolizes conviction. 3 is the first true male number, odd number, and symbolizes harmony. The first square number, 4, represents definition and revenge. The sum of the first number of women and men represents marriage. Seven was a particularly awe-inspiring subject for the Pythagoreans. Seven planets, or wandering stars, were awarded.
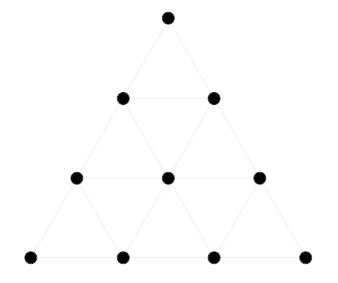 Tetractis
Tetractis
The most sacred of all was 10, or tetrachys. It symbolized the number of universes, and it was the sum of all the generative sources of geometric dimensions. 10 = 1+2+3+4, where element 1 represents a point (0-dimensional), and element 2 represents a line (1-dimensional, two points then a line). 3 represents a plane (2-dimensional, three points make a triangle and one plane is determined); 4 represents a three-dimensional (three-dimensional, four points make a cube tetrahedral). One of the great intellectual achievements of the Pythagorean school is that it deduced the special status of 10 through abstract mathematical discussions. The number 10 is not just the sum of two fingers. Ten is called the trigonometric number. trigonometric number means the number of triangles when the elements are drawn. The smaller triangular numbers are 3 and 6. The following trigonometric number of 10 is 15.
It is said that Philolaus, who led the post Pythagorean school, left a message about the cult of trigonometric number, especially Tetractis. He described the divine Tetractis as omnipotent, creating everything, the origin and guide of divine life and earthly life. Tetractis, like its name, has a four-integer square pyramid structure with four integers. Each number has a metaphysical meaning as well as a morphological meaning. The first integer, 1, is the monad, which represents the source of all things, one as a whole, and the principle of unity. The second integer, 2, is called "duad" or "dyad", which symbolizes duality, which means differentiation of all things. The third integer 3 is the triad, which controls and harmonizes the polarities of the undifferentiated monads and the duads that want to divide indefinitely. The first three dimensions, i.e. four tetrad, are the basis of matter.
The Pythagorean school thought that the purification and immortality of the soul were achieved through the study of mathematics and philosophy. They found a more pure lifestyle in scientific and mathematical reasons than anything else. They believed that Mathematical reasons liberate humans from delusion of objects, and lead to the number world eternal and orderly.
The Pythagorean school has discovered in music that mathematics and nature are closely related. First of all, they noted that music is deeply related to essence. They found that the string length of the instrument is proportional to the pitch it produces. For example, the pitch increases proportionally with the length of the string decreasing, and if the length is reduced in half, the pitch increases by one octave.
 Pythagoras and Philolaus, who test the notes of the instrument. A medieval woodblock print in Francino Gapurio's "Theory Music" (1492).
Pythagoras and Philolaus, who test the notes of the instrument. A medieval woodblock print in Francino Gapurio's "Theory Music" (1492).
As such, they saw that the pitch was expressed as integer ratio of a string or pipe-length. Therefore, for the Pythagorean school, music was a decisive proof of the fact that the world is full of numbers. Pythagoras recognizes that the sound from instruments such as the lyra and the flute depends on the length of the strings and pipes, and that their length is the best harmony to hear when they form a simple integer ratio.
From this, Pythagoras realized a close relationship between math and nature. For example, He confirmed that 1(Do) 8/9(Re) 64/81(Mi) 3/4(Fa) 2/3(Sol) 16/27(Ra) 128/243(Si) 1/2(Do). He also found that if you adjust the frequency of the strings to 24:27:30:32:36:40:45:48, the notes become a Do Re Mi Pa So la Si Do, and if you combine them, it creates a beautiful chord. They found evidence that the ratio of the frequency, or the ratio of the number, formed harmony. Pythagoras thought that numbers existed before all things came into being, numbers were considered the first things in nature, and that the whole was a smusical cale and a number.
<Author>